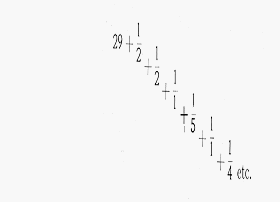|
Playing with continued fractions in Escher's garden Contents 1 introduction 1 Introduction Already as a child I was deeply interested in the products of frosty weather: frozen sproutstalks in the snowy kitchengarden, icicles hanging from the gutter, frozen puddles in the country-road behind my father's farm, the footprints of a hare in the snow and snowflakes on the window-sill. Of all of these, the snowflakes put the greatest spell on my imagination because of the mystery of arrangement that lay hidden in them. No one has ever put this 'hidden splendour' in better words than Thomas Mann in his Magic Mountain : | ||||||||


|


| "...and among these myriads of enchanting little stars, in their hidden splendor, too small for man's naked eye to see, there was not one like unto another; an endless inventiveness governed the development and unthinkable differentiation of one and the same scheme, the equilateral, equiangled hexagon. Yet each in itself - this was the uncanny, the antiorganic, the life-denying character of them all - each of them was absolutely symmetrical, icely regular in form." |
||||||
|
As I was growing older, my fascination reached a more abstract level. Now it were the principles of order and regularity in general, not necessarily in relation to frost, by which I was highly intrigued. I searched around in the field of crystallography, and found out how I could cause crystals to grow. I acquainted myself with the theory of mathematical groups (Speiser) and learned how to discriminate between the numerous types of symmetrical arrangement, the so-called 'symmetry groups'. Of these groups, I was especially interested in those 17 which can occur in the plane, the so-called 'wallpaper groups' . | ||||||||
| ||||||||
|
After some time my fascination became so strong that I began to perceive principles of arrangement
everywhere around me, in the streets, on elevator floors, even in the blouses of passers-by.
When on a social visit, my attention might focus more on the pattern of the wallpaper than on the conversation
around me. As to my ideals for the future, I dreamed of a life as an artist in abstract geometry,
one who does not add anything to Creation but who only makes its beauty manifest.
For I was convinced that great beauty lay hidden within the structures of perfect order.
It was just a matter of finding a method to unveil this hidden beauty. | ||||||||
| For centuries on end man had used symmetrical arrangements in the decoration of his buildings, clothes and furniture. Most often, the motif of a decoration had originated in the mind of the decorating craftsman. It was regularly repeated by him according to a certain pattern or arrangement. |  |
|||||||
But sometimes, the decorating artist would derive the shape of his motif from the constraints that are inherent in the arrangement itself. In such cases motif and arrangement are strongly interwoven. I knew of great predecessors in this field, in the distant past, but also contemporary. | ||||||||
- The Egyptian craftsmen of the days of the great Pharaohs are known to have been masters in this skill. Especially when spiral elements were introduced in symmetries in which reflections, slide-reflections and or rotations occured, the shape of the motif was to a large degree dictated by the constraints of the arrangement. |

 

| |||||||
- This interest in working with symmetry-constraints reached a culmination point during the prime time of Islamic culture, probably as a result of the Koranic injunction against the making of images. |
 | |||||||
- In our time, it was Maurits Escher who, deeply impressed by what these Islamic artists had brought forward, picked up the thread and astonished the world with his vertiginous tour de force by using the constraints of symmetrical structures in his work. | ||||||||
In the field of artfully dealing with symmetry I feel connected to all of these predecessors. To Maurits Escher I feel akin in an emotionally way. With him I share that feeling of loneliness which seems indissolubly linked with a fascination for regularity and order. Maurits Escher once put this feeling into words in his beautiful, expressive, somewhat megalomanic way: | ||||||||
| All alone, I walk in this magnificent garden which does not belong to me. Though its gate is wide open to everyone, I sojourn there in refreshing, but at the same time dejecting loneliness. I have, therefore, testified to the existence of this garden of Eden for many years... without expecting to attract many visitors. For the things by which I am truly fascinated and which I experience as beautiful are obviously considered dull and dry by others. | 
|
|||||||
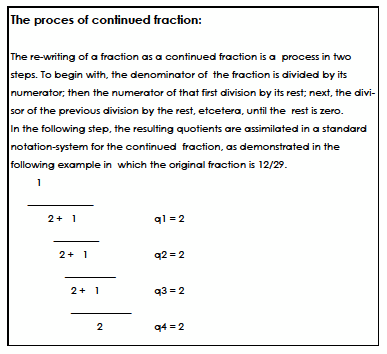
However, the practices of my predecessors gave me little help in pursuing my ideals. For in my opinion there was still too much personal freedom in the way in which they derived shapes from constraints. I wished to develop a decorational art in which simple arithmetical procedures, rooted in these constraints, determined the unfolding of the motif. While such was the ideal I was striving after, I had no idea how to bring this about. 2 Strips p.m. 3 Spirals and branches p.m. 4 Continued fraction In my attempts to solve this problem, I developed, all by myself, the principle of the continued fraction. It was 1983 by then. I was not aware, at the time, of the fact that this principle had already been known for centuries, and that it had been an important mathematical tool for clockmakers in designing the gear mechanism of clocks. | ||||||||
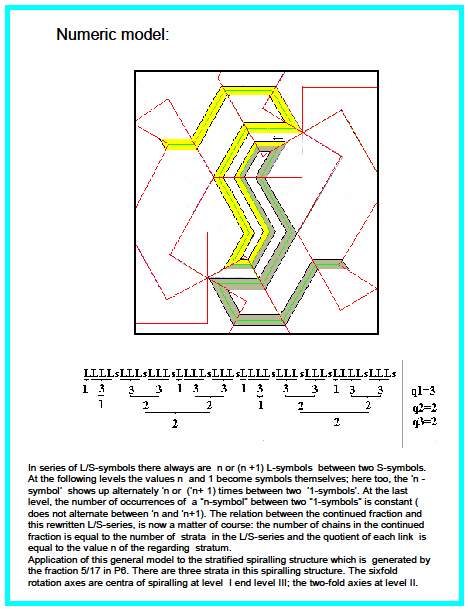
This continued fraction, because it, too, was stratified in character, was a better starting point for a mathematical clarification of the relation between the values in the fraction and the complexity of the strip-course. But to really clarify the relation between fraction and figure complexity, more was needed. The L/S series which represented the complexity of the strip-course also needed to be worked out in such a way that its stratified structure became explicit. If this is done in the right way, the relation between the two (i.e. the continued fraction and the L/S series) becomes a matter of course. In the beginning, however, I could not get it absolutely right, and as a result I was not able to interpret quotients with value 1 in the continued fraction (*). The problem kept me bothering like a smoulding piece of mischief which could, at certain times, turn into a roaring blaze. At long last I managed to solve it. It was not until 1993 that I managed to solve it. During a holiday, while on a trip to Hungary (obsessions do not slumber in holidays...), on a dingy little road along the river Donau, at the Austrian-Hungarian border, the solution suddenly came to me. Now that the relation between fraction and complexity of the strip-course had been clarified in a mathematical way, my mission had been, properly speaking, fulfilled. Yet, there was something which kept me uneasy: it was the notion that there must be a more fundamental way of implementing a fraction in a symmetry group, and that this way would have a direct link with the development of crystal faces. So, once again, I was at the starting point of a long and arduous adventure with an unpredictable outcome. (*) note: Initially I did not take n and 1 as symbols on abstracter levels, but n and (n-1). In continued fractions that possesed more than one link in succession in which q=1 (for instance 3/5 and 5/8) , this caused a lot of trouble. 5 Pattern generation based on Miller indices p.m. 6 Rainy sunday afternoons Several years have passed since, in matters of pattern generation, I have let bygones be bygones. The obsession which had a hold on my mind during a large part of my life is at long last lying doggo. It is only on rainy Sunday afternoons that my old passion may sometimes suddenly blaze up again in full force. At such moments I feel convinced that there is such immense beauty inherent in structures of perfect order that many an artist might wish that this beauty had originated in his own mind, instead of having been present in crystal structures from the beginning of time, long before Man came on earth to wonder at the marvel of it. |
||||||||
Long before there were people on earth, the crystals were already growing in the crust of the earth. One day a man saw such a sparkling little piece of regularity. He picked it up and looked at it as it lay in the palm of his hand and he marvelled at it. |

| |||||||